Higher Order Methods for Transitional and Turbulent Flows
The project uses direct numerical simulations (DNS) to study unsteady, incompressible transitional and turbulent flows of Newtonian and non-Newtonian fluids. The flows studied in this project are relevant to engineering applications such as fluid transport through pipelines and environmental fluid dynamics problems such as firewhirls and dust devils.
Area of science
Turbulent Flows Fluidisation and Fluid Mechanics Aerodynamics (excl. Hypersonic Aerodynamics)
Systems used
Magnus
Applications used
Semtex, OpenFoamThe Challenge
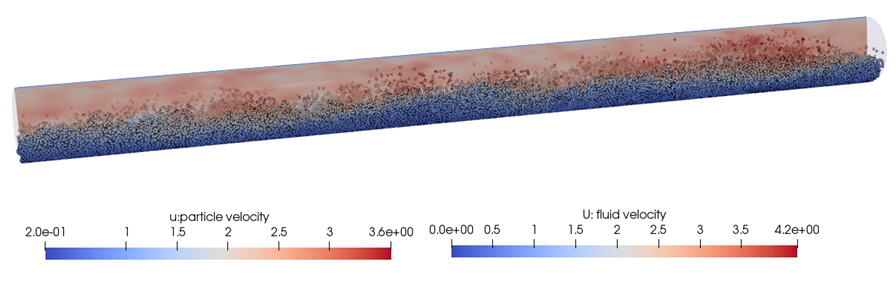
In one of the project, we simulate the laboratory scale firewhirls. Firewhirls are often encountered in bushfires and pose a danger to firemen as they significantly increase the fire spreading rate which is difficult to predict. In another project, we study the non-Newtonian turbulence in a pipe and the fate of particle suspension under the presence of a non-uniform viscosity of non-Newtonian fluids. In mining applications where the waste after mineral processing is transported to their disposal facilities via long pipelines, settling of the solid particles may clog the pipelines leading to the shutdown of the facility. We use DNS which although computationally very expensive but are free from the errors caused by turbulence models used in other less expensive computational techniques such as Reynolds-averaged Navier-Stokes equations and large-eddy-simulations.
The Solution
We carry out DNS and the stability analysis of model firewhirls to identify the critical flow parameters leading to their formation. This will help in improving the existing fire-spreading rate models. For particle suspension modelling, we carry out DNS-DEM (Discrete element modelling) simulations to identify the differences caused by the non-uniform viscosity of a non-Newtonian fluid to the fate of solid particle suspensions.
The Outcome
By taking advantage of the massive parallel structure of the DNS code (Semtex) and using Pawsey’s computational resources, we could significantly reduce the turn around time of the simulations.
As mentioned above, DNS are computationally very expensive as they resolve the smallest length scales in the flow. However, it has advantages over other less expensive computational techniques that unlike those techniques, DNS are free from the errors that may be caused by the turbulence models. Even for Newtonian fluids, the existing turbulence models are not reliable and may lead to errors in flow predictions. Non-Newtonian fluids further increase the complexity in governing equations due to their shear-dependent non-uniform viscosity. By taking advantage of the massively parallel structure of the simulations codes (Semtex and OpenFOAM) and Pawsey’s computational resources, we are significantly reducing the computational turn-around time to make realisable progress in real time.
List of Publications
The following are the publications in 2018 from the current project:
1. J. Singh, M. Rudman and H. M. Blackburn, “Reynolds number effects in pipe flow turbulence of generalized Newtonian fluids,” Physical Review Fluids, vol. 03, pp. 094607,2018
2. Enzu Zheng, M. Rudman, J. Singh, and Shibo Kuang, “Direct Numerical Simulation of Turbulent Non-Newtonian Flow using OpenFOAM,“ under review for publication in Appl. Math. Model.
3. J.Singh, H.M.Blackburn, J.M.Lopez, A.J.Smits, “Linear stability analysis and direct numerical simulations of buoyant and non-buoyant columnar vortices,” under preparation for J. Fluid. Mech.
4. J. Singh, H. M. Blackburn, J. M. Lopez, A. J. Smits, “Numerical Investigation of Swirling Buoyant Flows Relevant to Fire Whirls and Dust Devils,” in 21st Australasian Fluid Mech. Conf., Adelaide, Australia, 2018
5. Enzu Zheng, M. Rudman, J. Singh, and Shibo Kuang, “Assessing OpenFOAM for DNS of Turbulent Non-Newtonian Flow in a Pipe,“ in 21st Australasian Fluid Mech. Conf., Adelaide, Australia, 2018

Figure 1. Shape of the leading eigen mode of different azimuthal wavenumbers visualised via the iso-surfaces of axial vorticity for an inlet swirl angle of 45 deg with base heating characterised by Richardson number Ri=1.4 at a Reynolds number Re=500.
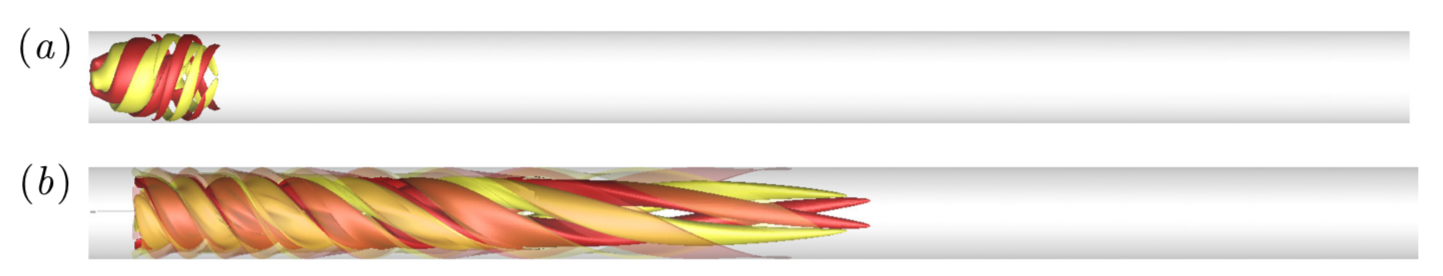
Figure 2. Iso-surfaces of the axial vorticity in DNS plotted for (a) a flow with no heating and (b) with heating characterised by Richardson number Ri=1.4 at Reynolds number Re=500 and inlet swirl angle of 50 deg. Much longer structures are seen in the flow with heating compared to the non-heating case.